how to find the volume of a rectangle
This folio explains how to summate the volume of solid objects, i.due east. how much you could fit into an object if, for example, you lot filled it with a liquid.
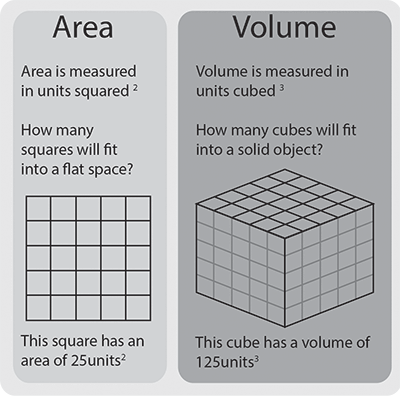
Area is the measure out of how much space at that place is within a two dimensional object (see our page: Computing Area for more).
Book is the measure out of how much space there is within a 3-dimensional object. Our folio on 3-dimensional shapes explains the basics of such shapes.
In the real world, calculating volume is probably not something that yous will use as often as calculating area.
However it tin can still exist of import. Being able to summate volume will enable you lot to, for example, piece of work out how much packing space y'all have when moving house, how much office space y'all need, or how much jam you can fit into a jar.
It tin can besides be useful for understanding what the media mean when they talk about the capacity of a dam or the flow of a river.
A Note on Units
Area is expressed in square units (two), because it is it is measured in two dimensions (due east.g. length × width).
Book is expressed in cubic units (three), considering information technology is measured in three dimensions (east.g. length × width × depth). Cubic units include cm3, m3 and cubic anxiety. Cubic units include cm3, chiliad3 and cubic feet.
Alarm!
Volume tin also exist expressed as liquid capacity.
Metric Arrangement
In the metric system liquid capacity is measured in litres, which is straight comparable with the cubic measurement, since 1ml = 1cmthree. 1 litre = 1,000 ml = ane,000cm3.
Imperial/English Organization
In the royal/English system the equivalent measurements are fluid ounces, pints, quarts and gallons, which are not easily translated into cubic feet. Information technology is therefore best to stick to either liquid or solid book units.
For more, come across our page on Systems of Measurement.
Basic Formulae for Calculating Volume
Volume of Rectangle-Based Solids

Whereas the bones formula for the area of a rectangular shape is length × width, the basic formula for volume is length × width × tiptop.
How you refer to the dissimilar dimensions does non change the calculation: y'all may, for example, use 'depth' instead of 'height'. The important thing is that the three dimensions are multiplied together. You can multiply in which-always order you similar as it won't change the answer (see our folio on multiplication for more).
A box with the dimensions 15cm width, 25cm length and 5 cm acme has a book of:
15 × 25 × 5 = 1875cm3
Volume of Prisms and Cylinders
This basic formula can be extended to cover the volume of cylinders and prisms too. Instead of a rectangular cease, you lot simply have another shape: a circle for cylinders, a triangle, hexagon or, indeed, whatever other polygon for a prism.
Finer, for cylinders and prisms, the book is the area of one side multiplied by the depth or height of the shape.
The bones formula for volume of prisms and cylinders is therefore:
Expanse of the stop shape × the height/depth of the prism/cylinder.
Watch out for inconsistent units!
A direct length of round piping has an internal bore of 2cm and a length of 1.7m. Calculate the volume of h2o in the pipe.
In this example you need to calculate the volume of a very long, sparse cylinder, that forms the inside of the piping. The area of i finish can exist calculated using the formula for the expanse of a circumvolve πrii. The bore is 2cm, so the radius is 1cm. The area is therefore π × one2, which is 3.14cm2.
The length of the pipe is 1.7m, and so you need to multiply the end expanse by the length in social club to detect the volume.
Lookout out for inconsistent units! The area is in centimetres, but the length is in metres. First convert the length into cm 1.7 × 1000 = 1700cm.
The volume is therefore 3.14 × 1700 = 5338 cmthree. This is equivalent to 5.338 litres, or 0.0053 m3.
Volume of Cones and Pyramids
The same principle every bit higher up (width × length × height) holds for computing the book of a cone or a pyramid except that, considering they come to a signal, the volume is only a proportion of the total that it would be if they continued in the aforementioned shape (cross-department) right through.
The volume of a cone or pyramid is exactly 1 third of what it would be for a box or cylinder with the same base.
The formula is therefore:
Area of the base of operations or end shape × the peak of the cone/pyramid × 1/iii
Refer dorsum to our page Calculating Area if yous cannot call up how to calculate the area of a circumvolve or triangle.
For case, to summate the volume of a cone with a radius of 5cm and a height of 10cm:The area within a circle = πrii (where π (pi) is approximately three.xiv and r is the radius of the circle).
In this example, area of base (circumvolve) = πr2 = iii.14 × 5 × 5 = 78.5cmtwo.
78.5 × 10 = 785
785 × 1/3 = 261.6667cm3

Volume of a Sphere
Equally with a circumvolve, you demand π (pi) to calculate the volume of a sphere.
The formula is four/3 × π × radius3.
You lot may be wondering how you could work out the radius of a ball. Curt of sticking a knitting needle through information technology (constructive, but terminal for the brawl!), there is a simpler way.
You can mensurate the distance around the widest point of the sphere directly, for example, with a record measure. This circle is the circumference and has the same radius as the sphere itself.
The circumference of a circle is calculated equally 2 10 π x radius.
To calculate the radius from the circumference yous:
Divide the circumference by (2 x π).
Worked Examples: Computing Volume
Example 1

Calculate the volume of a cylinder with a length of 20cm, and whose circular terminate has a radius of 2.5cm.
First, work out the area of one of the circular ends of the cylinder.
The area of a circumvolve is πr2 (π × radius × radius). π (pi) is approximately 3.14.
The expanse of an end is therefore:
3.fourteen x 2.five ten 2.v = nineteen.63cm2
The volume is the area of an end multiplied past the length, and is therefore:
xix.63cmii x 20cm = 392.70cm3

Instance ii
Which is bigger by book, a sphere with radius 2cm or a pyramid with base of operations 2.5cm foursquare and tiptop of 10cm?
First, work out the volume of the sphere .
The book of a sphere is iv/3 × π × radiusiii.
The book of the sphere is therefore:
4 ÷ three ten 3.fourteen × ii × 2 × two = 33.51cmthree
Then work out the volume of the pyramid .
The book of a pyramid is 1/three × area of base × acme.
Area of base = length × breadth = ii.5cm × 2.5cm = 6.25cm2
Volume is therefore i/3 10 6.25 × 10 = 20.83cm3
The sphere is therefore larger by volume than the pyramid.
Calculating the Volume of Irregular Solids
Just as you tin calculate the area of irregular ii-dimensional shapes past breaking them downwards into regular ones, you can do the same to calculate the volume of irregular solids. Simply split the solid up into smaller parts until you reach only polyhedrons that you can work with easily.
Worked example
Calculate the book of a water cylinder with total acme 1m, bore of 40cm, and whose height department is hemispherical (half of a sphere).

You first dissever the shape into two sections, a cylinder and a hemisphere.
The volume of a sphere is 4/3 × π × radiusthree. In this case the radius is 20cm (half the bore). Because the top is hemispherical, its book volition be one-half that of a total sphere. The volume of this section of the shape therefore:
0.5 × iv/three × π × 203 = sixteen,755.16cm3
The volume of a cylinder is area of the base × peak. Here, the meridian of the cylinder is the total elevation less the radius of the sphere, which is 1m – 20cm = 80cm. The area of the base is πr2.
The volume of the cylindrical department of this shape is therefore:
80 × π × 20 × xx = 100,530.96cmthree
The total volume of this water container is therefore:
100,530.96 + 16,755.sixteen = 117,286.12cm3.
This is quite a large number, so y'all may prefer to catechumen it to 117.nineteen litres by dividing past one,000 (since in that location are 1000cmiii in a litre). However, it is quite right to express it every bit cmiii since the problem does not enquire for the answer to be expressed in any item form.

Further Reading from Skills Y'all Need
Understanding Geometry
Role of The Skills You Need Guide to Numeracy
This eBook covers the basics of geometry and looks at the properties of shapes, lines and solids. These concepts are congenital up through the volume, with worked examples and opportunities for you to practise your new skills.
Whether y'all want to brush up on your basics, or help your children with their learning, this is the book for y'all.
In Determination…
Using these principles, if necessary, you should at present exist able to calculate the book of nearly anything in your life, whether that'southward a packing crate, a room, or a water cylinder.
Source: https://www.skillsyouneed.com/num/volume.html
Posted by: cummingstheplain.blogspot.com

0 Response to "how to find the volume of a rectangle"
Post a Comment