how do you find the square root of a number

Square root of a number is a value, which on multiplication past itself gives the original number. The foursquare root is an inverse method of squaring a number. Hence, squares and foursquare roots are related concepts.

Suppose, x is the square root of y, then it is represented as x=√y or nosotros tin express the same equation as x ² = y. Here,'√'is the radical symbol used to correspond the root of numbers. The positive number, when multiplied past itself, represents the foursquare of the number . The square root of the square of a positive number gives the original number.

For case, the square of 3 is 9, 3² = ix and the foursquare root of 9, √9 = 3. Since 9 is a perfect foursquare, hence it is easy to observe the square root. Merely for an imperfect square like 3, 7, 5, etc., we accept to use different methods to detect the square root. Acquire square roots from 1 to 25 with some shortcut tricks hither.

Table of Contents:

Definition
Symbol
Formula
Properties
Finding Square Root
- By Prime Factorisation
- By Repeated Subtraction Method
- By Long Division Method
- Past Estimation Method
Perfect Squares
Square Root Table
Square root of decimal
Square root of negative number
Foursquare Root of Complex Numbers
Square Root Equation
Square of a Number
Applications
List of Square roots
Solved Problems
Worksheet
FAQs

Definition of Square Root

The square root of whatever number is equal to a number, which when squared gives the original number.
Allow u.s.a. say g is a positive integer, such that √(one thousand.m) = √(yard²) = thou

In mathematics, a foursquare root office is divers every bit a one-to-one part that takes a positive number as an input and returns the square root of the given input number.

f(x) = √x

For instance, if 10=4, then the part returns the output value equally 2.

Note: The square root of a negative number represents a complex number.

Suppose √-north = i√n, where i is the imaginary number.

Square Root Symbol

The square root symbol is usually denoted as ' √' . It is called a radical symbol. To correspond a number '10' as a square root using this symbol can be written as:
' √x '

where 10 is the number. The number under the radical symbol is called the radicand. For case, the square root of 6 is also represented equally radical of half dozen. Both represent the same value.

Square Root Formula

The formula to find the foursquare root is:

Since, y.y = y² = a; where 'a' is the square of a number 'y'.

Backdrop of Square root

Some of the important properties of the square root are as follows:

If a number is a perfect square number, and so at that place exists a perfect square root.
If a number ends with an even number of zeros (0'south), then information technology tin can have a square root.
The two square root values can be multiplied. For example, √iii can be multiplied by √two, then the outcome should be √6.
When two aforementioned square roots are multiplied, and then the result should be a radical number. Information technology means that the result is a non-foursquare root number. For example, when √vii is multiplied by √7, the result obtained is 7.
The foursquare root of any negative numbers is not defined. Because the perfect square cannot be negative.
If a number ends with 2, 3, 7 or eight (in the unit digit), then the perfect square root does not exist.
If a number ends with 1, iv, 5, half-dozen or 9 in the unit digit, and then the number volition accept a square root.

How do Notice Square Root of Numbers?

To notice the square root of any number, we need to effigy out whether the given number is a perfect foursquare or an imperfect square. If the number is a perfect square, such as 4, 9, 16, etc., then we tin can factorize the number by prime factorisation method. If the number is an imperfect foursquare, such as two, 3, 5, etc., then we have to use a long division method to find the root.

Hence, the methods to find the foursquare root of numbers are:

Foursquare Root past Prime Factorisation
Square Root by Repeated Subtraction Method
Square Root by Long Division Method
Foursquare Root past Estimation Method

Allow us learn each method of square root with examples.

Square root By Prime number Factorisation

The square root of a perfect square number is easy to calculate using the prime factorisation method. Let us solve some of the examples here:

Number	Prime Factorisation	Square Root
16	2x2x2x2	√16 = 2×2 = 4
144	2x2x2x2x3x3	√144 = 2x2x3 = 12
169	13×xiii	√169 = thirteen
256	256 = 2×two×two×2×2×2×ii×two	√256 = (2x2x2x2) = 16
576	576 = 2x2x2x2x2x2x3x3	√576 = 2x2x2x3 = 24

Square Root past Repeated Subtraction Method

As per the repeated subtraction method, if a number is a perfect square, then we can make up one's mind its square root by:

Repeatedly subtracting consecutive odd numbers from it
Subtract till the difference is cypher
Number of times we subtract is the required foursquare root

For case, let us discover the square root of 25.

25 – 1 = 24
24 – three = 21
21 – 5 = xvi
16 – 7 = 9
9 – nine = 0

Since, the subtraction is done for 5 times, hence the square root of 25 is 5.

Foursquare Root by Long Sectionalisation Method

Finding square roots for the imperfect numbers is a fleck hard just we can calculate using a long segmentation method. This can be understood with the help of the case given below. Consider an case of finding the square root of 436.

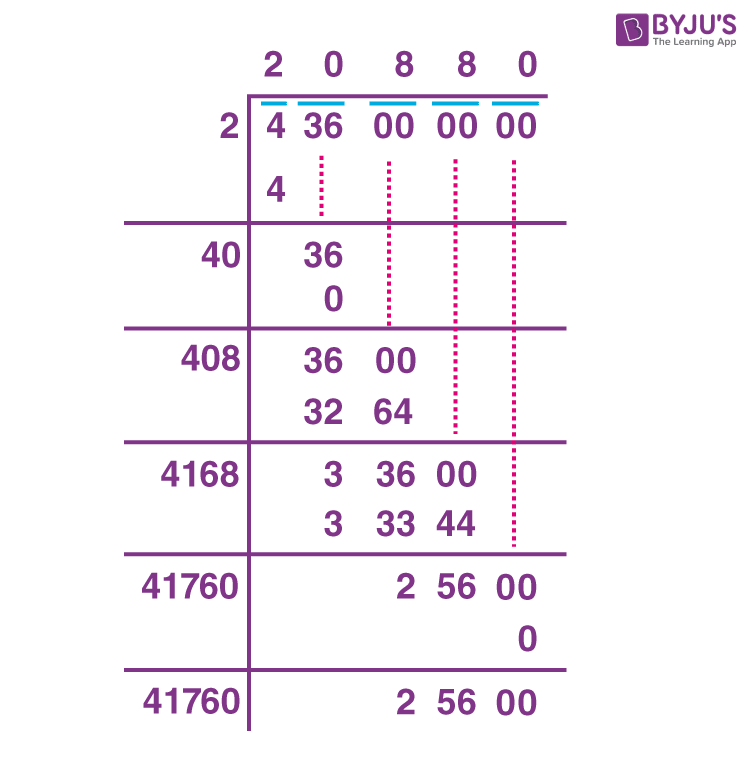
Square root by long division method

Thus, the square root of 436 is 20.880 (rounding to iii decimals).

Square root by Estimation Method

This method is used every bit an approximation method, to find the foursquare root past guessing the values.

For example, the square root of 4 is two and the square root of 9 is 3, thus we can guess, square root of 5 will prevarication betwixt two and 3.

But, we need to check the value of √v is nearer to two or 3. Let us find the square of two.two and 2.8.

2.2² = 4.84
ii.8² = seven.84

Since the square of two.two gives an approximate value of 5, thus we can guess the square root of 5 is equal to 2.2 approximately.

How to Find Square Roots Without Calculator?

This is quite an interesting way to effigy out the square root of a given number. The process is completely based on the method called "guess and cheque". Guess your answer, and verify. Repeat the procedure until you have the desired accurate result. We can also use the long division method to discover the foursquare root of a number.

Square Root of Perfect squares

Beneath are the numbers which are perfect squares and then finding the square roots of such numbers is easy.

i² = 1⇔ √1 = i
two² = 4⇔ √iv = 2
three² = 9⇔ √9 = iii
fourⁱⁱ = 16⇔ √xvi = 4
5² = 25⇔ √25 = 5
half dozen² = 36⇔ √36 = 6
vii² = 49⇔ √49 = 7
eight² = 64⇔ √64 = eight
9² = 81⇔ √81 = 9
10² = 100⇔ √100 = x

Hence, i, 4, 9, xvi, 25, 36, 49, 64, 81 and 100 are the perfect squares here. Check square roots of some numbers hither:

Square Root Tabular array (i to 50)

Hither is the list of the foursquare root of numbers from 1 to 50.

√due north	Value	√n	Value	√n	Value
√1	one	√18	4.2426	√35	five.9161
√2	1.4142	√19	iv.3589	√36	6
√3	1.7321	√20	four.4721	√37	half dozen.0828
√4	2	√21	four.5826	√38	6.1644
√5	2.2361	√22	four.6904	√39	6.2450
√6	2.4495	√23	4.7958	√40	vi.3246
√7	2.6458	√24	four.8990	√41	6.4031
√8	two.8284	√25	5	√42	six.4807
√nine	iii	√26	five.0990	√43	six.5574
√10	3.1623	√27	5.1962	√44	6.6332
√11	3.3166	√28	5.2915	√45	6.7082
√12	3.4641	√29	5.3852	√46	6.7823
√13	3.6056	√30	five.4772	√47	6.8557
√14	3.7417	√31	5.5678	√48	six.9282
√15	three.8730	√32	5.6569	√49	seven
√16	4	√33	5.7446	√l	seven.0711
√17	4.1231	√34	5.8310

Foursquare Root of Decimal

A decimal value volition take a dot (.) such as 3.viii, 5.2, 6.33, etc. For a whole number, nosotros have understood how to derive the foursquare root simply permit us see how to get the square root of a decimal.

Example: Notice the foursquare root of 0.09.Allow N² = 0.09

Taking root on both sides.

N = ±√0.09

Every bit we know,

0.3 x 0.3 = (0.3)² = 0.09

Therefore,

Northward = ±√(0.3)²

N = ±(0.3)

Square Root of Negative Number

The square root of a negative number is not a existent number but a complex number. It is because the foursquare of any integer is a positive value. The square root of a negative number, say, -y, is: √(-y)= i√y, where 'i' is the square root of -one.

Square root of Complex Numbers

To find the square root of complex numbers is a footling complicated. We tin can observe the foursquare root of a+ib using the below formula:

\(\begin{array}{l}\sqrt{a+b i}=\pm(\sqrt{\frac{\sqrt{a^{2}+b^{2}+a}}{2}}+i \sqrt{\frac{\sqrt{a^{ii}+b^{two}-a}}{ii}})\stop{assortment} \)

where a+ib is a complex number.

How to Solve the Square Root Equation?

A square root equation is such an equation that has a variable in the radicand of the root. It is as well called the radical equation.

To solve the radical equation, we need to follow the below steps:

Isolate the foursquare root to ane of the sides (L.H.S or R.H.Southward).
Foursquare both the sides of the given equation
Now solve the rest equation.

Let us empathise the steps with examples.

Instance: Solve the radical equation √(4a+9) – v = 0Solution: Given, √(4a+ix) – 5 = 0

Isolate the square root term first. Then the equation becomes,

√(4a+9) = 5

At present on squaring both the sides, we become; 4a+9 = 5²
4a + ix = 25

4a = 16

a = sixteen/4

a = iv

Condition 1: If the equation has more i radical or foursquare root.

If the radical equation has more than one root and then repeat the above given steps for each square root.

Case: Solve √(2x−5) − √(x−1) = 1 Let the states isolate ane of the square root.

√(2x−5) = 1 + √(10−one)

Now squaring both the sides

2x – 5 = (i + √(x−1)) ²

Utilise the algebraic identity, (a+b) ² = a ⁱⁱ + b ^two + 2ab.

2x−5 = 1 + 2√(x−i) + (10−ane)

2x−5 = 2√(ten−1) + 10

x−v = 2√(x−i)

Now again isolate the foursquare root.

√(ten−1) = (x−5)/2

x−i = ((10−5)/2) ^two

x−1 = (10 2 − 10x + 25)/iv

4x−4 = x ² − 10x + 25

4x − iv − x ⁱⁱ + 10x − 25 = 0

−x ^two + 14x − 29 = 0

x ² − 14x + 29 = 0

Using the quadratic formula, nosotros can solve the to a higher place equation.

ten = 2.53 and x = eleven.47

Squaring a Number

To find the foursquare of a number, we need to multiply the number past itself.

For example, two multiplied by 2 is equal to 4

Below is a 2 by ii tabular array that shows, a total of four blocks.

Similarly,

square of 5 is: 5 multiplied by 5 = five x 5 = five 2 = 25

Square of ix = ix two = ix x 9 = 81

Square of 15 = xv 2 = 15 x 15 = 225

Applications of Square Roots

The foursquare root formula is an of import department of mathematics that deals with many applied applications of mathematics and it too has its applications in other fields such as computing. Some of the applications are:

Quadratic equations
Algebra
Geometry
Calculus

List of Square Roots of Numbers

Video Lessons on Square Roots

Visualising square roots

Finding Square roots

Solved Examples of Foursquare Roots

Let usa empathize this concept with the help of an case:

Example one: Solve √10 to two decimal places.

Solution:

Stride one: Select whatsoever two perfect square roots that you feel your number may fall in betwixt.

We know that 2² = 4; iiiⁱⁱ = 9, 4² = 16 and five^two = 25

Now, choose 3 and four (as √10 lies between these two numbers)

Footstep two: Divide the given number by one of those selected square roots.

Split 10 past 3.

=> 10/3 = 3.33 (circular off respond at 2 places)

Step iii: Find the average of root and the result from the above pace i.e.

(3 + 3.33)/2 = 3.1667

Verify: iii.1667 10 iii.1667 = 10.0279 (Not required)

Repeat footstep 2 and step 3

At present 10/3.1667 = 3.1579

Average of iii.1667 and 3.1579.

(3.1667+3.1579)/ii = 3.1623

Verify: iii.1623 x iii.1623 = 10.0001 (more than authentic)

Cease the procedure.

Example ii: Find the square roots of whole numbers which are perfect squares from 1 to 100.

Solution: The perfect squares from i to 100: one, four, 9, 16, 25, 36, 49, 64, 81, 100

Square root	Event
√one	1
√4	ii
√9	three
√16	four
√25	5
√36	6
√49	vii
√64	8
√81	ix
√100	x

Example iii: What is:

The square root of 2
The square root of 3
The foursquare root of four
The square root of five

Solution: Use square root listing, we have

value of root 2 i.e. √ii = 1.4142
value of root three i.eastward. √3 = 1.7321
value of root 4 i.e. √4 = 2
value of root 5 i.east. √v = 2.2361

Example iv: Is square Root of a Negative Number a whole number?

Solution: No, Equally per the square root definition, negative numbers shouldn't have a square root. This is considering if we

multiply two negative numbers, the result volition ever exist a positive number. Square roots of negative numbers expressed

equally multiples of i (imaginary numbers).

Instance 5: Solve the equation: √(ten+ii) = 4

Solution: Given,

√(x+2) = iv

Squaring both the sides, we become;

x+two = √iv

10+2 = ±4

x = ±4 – 2

Therefore,

ten = 2 or x = -6

Practise Questions on Square roots

What is square root of 64?
Simplify √142
Discover the square root of 250
What is the square root of 10000?
Find the value of √12.
Are √155, √121 and √139 perfect squares?

Frequently Asked Questions on Square root

What is a foursquare root in Maths?

A foursquare root is a value that gives the original number when multiplied by itself.

How to find foursquare root?

To find the square root, we tin can use four methods: Prime number factorisation, Repeated Subtraction, Long sectionalization and Estimation method.

What is the meaning of this symbol '√'?

'√' is a radical symbol used to announce foursquare root of a number.

What are squares and square roots?

The square root is just the opposite process of squaring a number. Suppose nosotros square a number n, such equally n^2, then the foursquare root of due north^2 is equal to the original number n.

How to find the foursquare root of perfect squares?

By using prime factorisation, we can find the square root of perfect squares.

How to detect the foursquare root of imperfect squares?

Past using the long segmentation method, we can find the square root of imperfect squares.

To larn more about square roots and other Maths topics in a more than engaging and effective mode, register with BYJU'S- The Learning App.

Source: https://byjus.com/maths/square-root/

Posted by: cummingstheplain.blogspot.com