How To Find Terminal Point On Unit Circle
Concluding POINTS ON THE UNIT CIRCLE
Suppose t is a real number. Let'south marker off a distance t along the unit circumvolve, starting at the point (i, 0) and moving in a counterclockwise management, if t is positive or in a clockwise direction, if t is negative.
In this way we arrive at a betoken P(x, y) on the unit of measurement circle. The betoken P(x, y) obtained in this mode is called the terminal point determined past the existent number t.
Terminal Point P(x, y) Determined by t > 0 :
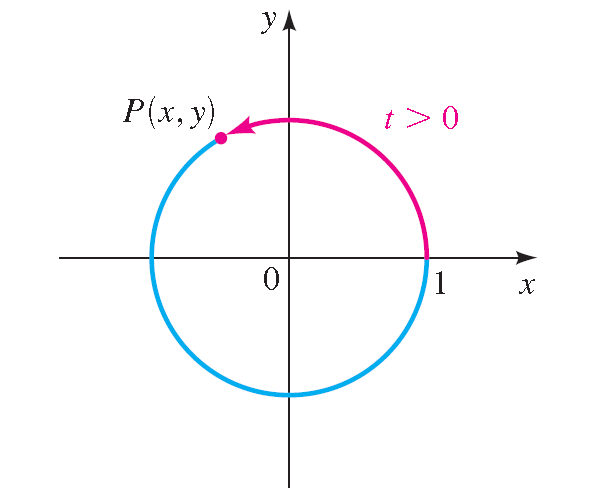
Terminal Betoken P(x, y) Determined by t < 0 :

The circumference of the unit circumvolve is
C = 2π(1) = 2 π
So, if a point starts at (ane, 0) and moves counterclockwise all the manner around the unit circle and returns to (one, 0), it travels a distance of 2π. To move halfway around the circle, it travels a altitude of (1/two)(2π) = π.
To motility a quarter of the distance effectually the circumvolve, information technology travels a distance of (1/4)(2π) = π/2. Where does the bespeak terminate up when it travels these distances along the circle? In the diagram shown below, we encounter, for example, that when it travels a distance ofπ starting at (1, 0), its final point is (-1, 0).
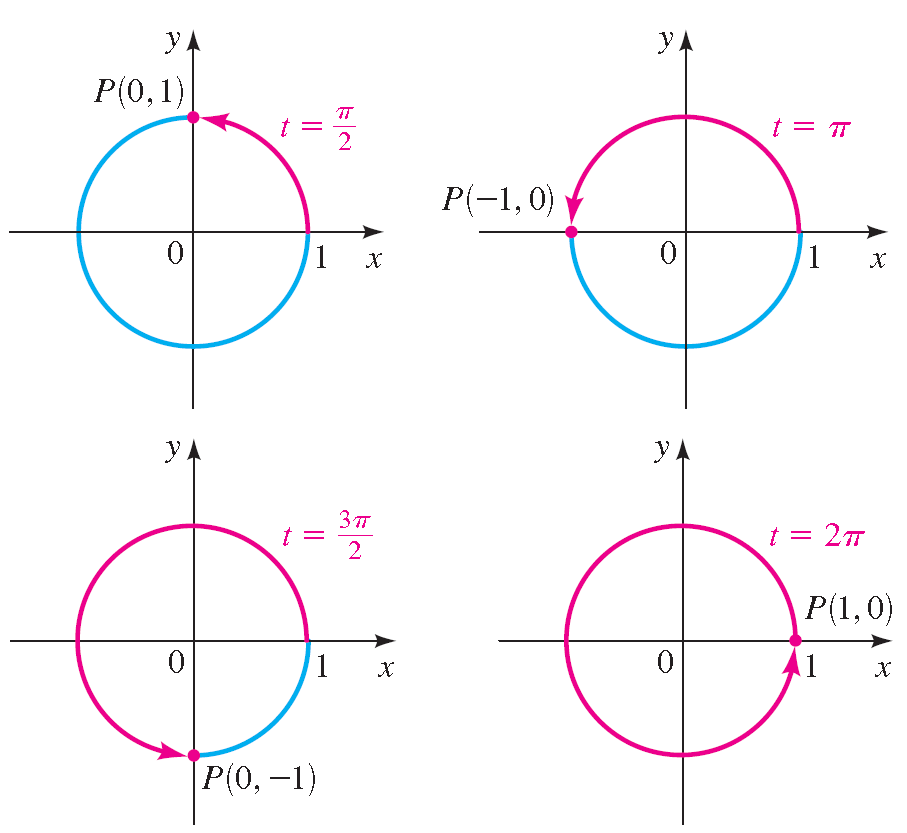
Finding Terminal Points
Observe the terminal betoken on the unit of measurement circle determined past each real number t.
Example 1 :
t = 3π
Solution :
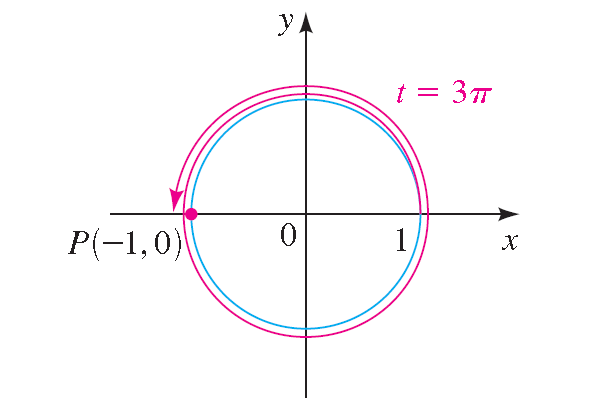
The terminal indicate determined by 3π is (-1, -0).
Example ii :
t = -π
Solution :
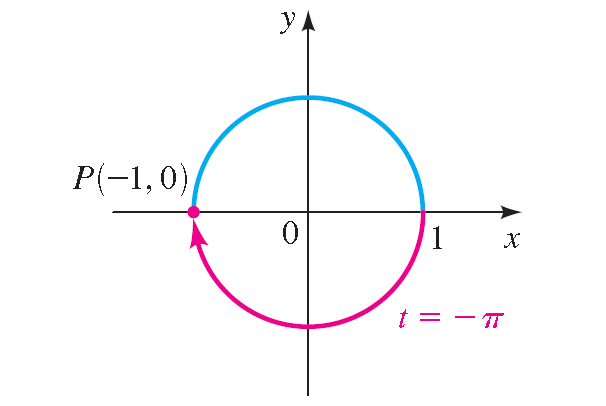
The terminal betoken determined past -π is (-ane, -0).
Example 3 :
t = -π/ii
Solution :
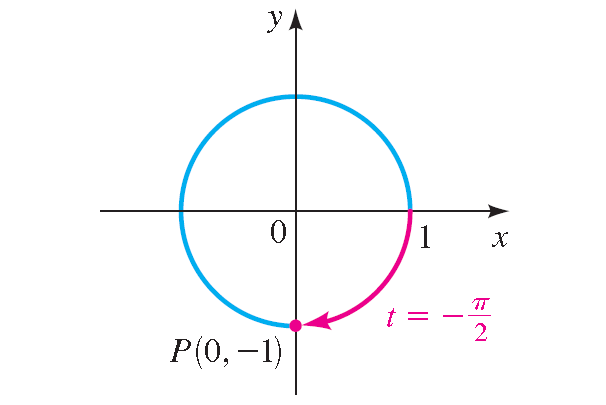
The terminal betoken determined by -π/2 is (0, -1).
Detect that different values of t tin can determine the same terminal signal.
The terminal point P(x, y) determined by t =π/iv is the same distance from (1, 0) equally from (0, 1) along the unit circumvolve.

Since the unit circle is symmetric with respect to the line y = x, it follows that P lies on the line y = x. So P is the signal of intersection (in the first quadrant) of the circlex 2 + y 2 = one and the line y = x.
Substituting x for y in the equation of the unit circumvolve.
10 2 + x ii = 1
2xii = ane
x2 = 1/2
k = ± 1/√2
Because P is in the kickoff quadrant, x = 1/√2 and y = x, we have y = 1/ √ 2 also.
Thus the terminal point determined by π/four is
P(i/ √ ii, 1/ √ 2) = P( √ 2/2, √ 2/2)
Like methods tin can be used to discover the final points determined by t = π/half dozen and t = π/3.
Table :
| t 0 π/6 π/4 π/3 π/2 | Terminal point adamant by t (1, 0) (√3/2, 1/2) (√two/2, √2/2) (one/2, √iii/ii) (0, 1) |
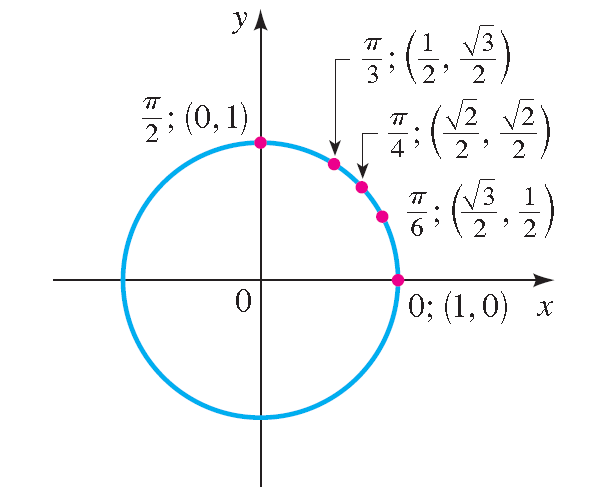
Example 4 :
t = -π/4
Solution :
Permit P be the terminal bespeak determined by - π/four, and let Q be the final point determined by π/4. In the diagram shown beneath, we see that the betoken P has the same coordinates as Q except for sign.
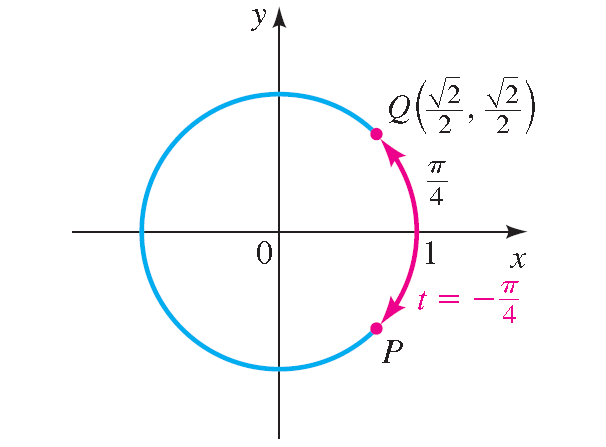
Because P is in quadrant 4, its x-coordinate is positive and its y-coordinate is negative. Thus, the terminal signal is
P( √2/two, - √2/2)
Case v :
t = 3π/4
Solution :
Permit P be the terminal point determined by 3 π/iv, and allow Q be the terminal point determined by π/four. In the diagram shown beneath, we meet that the indicate P has the aforementioned coordinates equally Q except for sign.
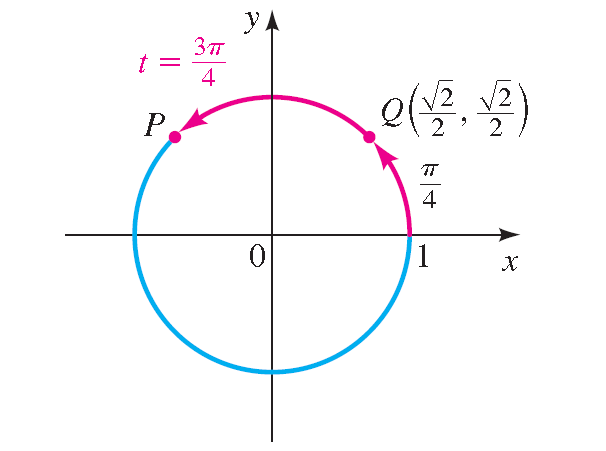
ConsideringP is in quadrant Two, its ten-coordinate is negative and its y-coordinate is positive. Thus, the terminal point is
P(-√two/two, √2/2)
Example 6 :
t = -5π/half dozen
Solution :
Let P exist the last bespeak adamant by -5 π/6, and let Q be the concluding signal determined past π/half-dozen. In the diagram shown below, nosotros run across that the bespeak P has the same coordinates every bit Q except for sign.
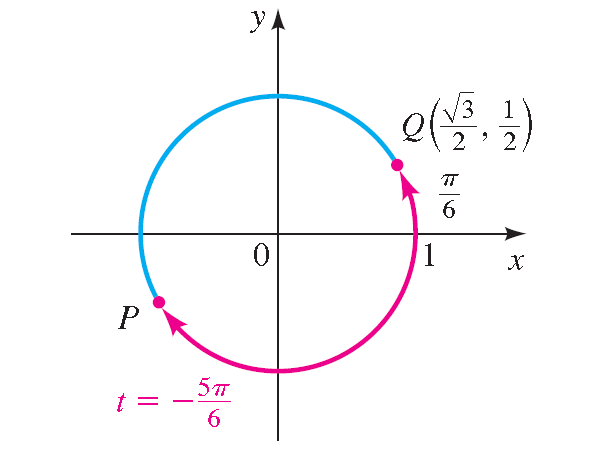
Because P is in quadrant Three, its both x-coordinate and y-coordinate are negative. Thus, the final betoken is
P(- √3/2, -1 /2)
Related Stuff
1. The Unit Circle
two. Reference Number on the Unit of measurement Circle
3. Using Reference Number to Notice terminal Points

Autonomously from the stuff given above, if you need whatever other stuff in math, please use our google custom search here.
Kindly post your feedback tov4formath@gmail.com
We e'er appreciate your feedback.
© All rights reserved. onlinemath4all.com
Source: https://www.onlinemath4all.com/terminal-points-on-the-unit-circle.html
Posted by: cummingstheplain.blogspot.com


0 Response to "How To Find Terminal Point On Unit Circle"
Post a Comment