How To Find The Domain Of A Fraction

We deal with fractions every day. Simply what exactly is a fraction? How do we get to know them better? In this tutorial we will explore the basics and practice together, then fractions tin become valuable helpers in everyday life and across.
Let's imagine a whole pie divided into iv equal parts. One part is shaded crimson.
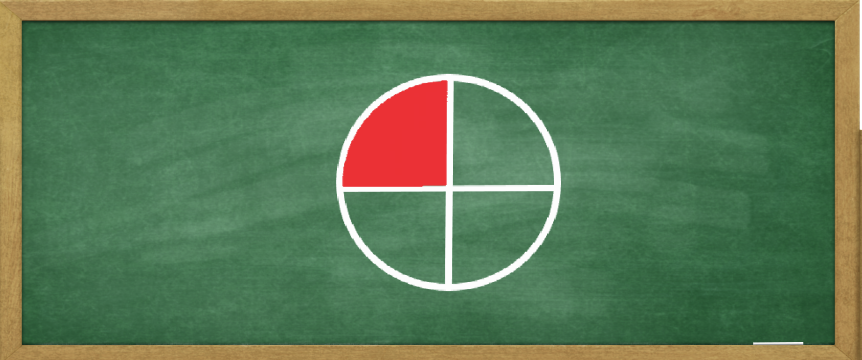
I red part out of four equal parts ways i/4 of a whole is shaded. If we call up of equal parts of a whole equally shares, one share of a pie here is shaded red.
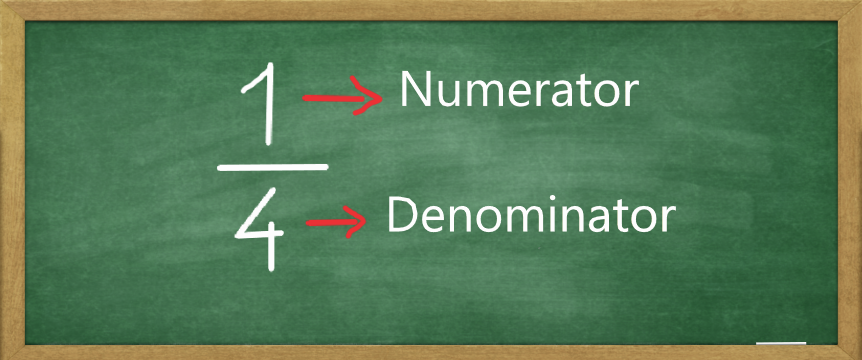
The number one above the line is chosen a Numerator. It shows how many shares are shaded. The number 4 below the line is called a Denominator. Information technology shows how many equal shares a whole is divided into. Allow's look at another example.
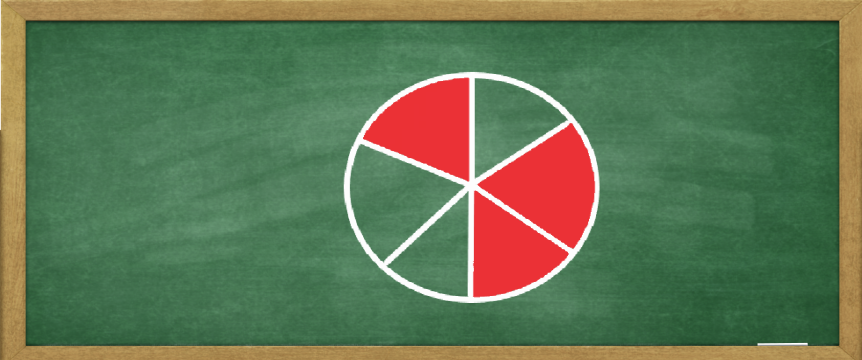
The new pie in a higher place is divided into vi equal shares. Therefore, the denominator will equal vi. Out of these half dozen equal shares 3 are shaded red. Therefore, the numerator volition equal 3. In other words, 3/6 of the pie is shaded.
At present allow's test what we have learned so far. As you know, there are 24 hours in a whole 24-hour interval. If you lot spent six hours studying, what fraction of the day did y'all spend studying?
What fraction of a twenty-four hours is 6 hours?
Choose 1 reply
A day is divided into 24 equal shares chosen hours. So the Denominator will be 24. Think of the 6 hours spent studying as 6 shaded shares of the pie. That volition make the Numerator equal 6. The fraction we are looking for is 6/24.
Part 2. Simplifying fractions
Retrieve the pie from previous case? It had iii/6 of it shaded reddish. Allow's add two new pies and look at them together.
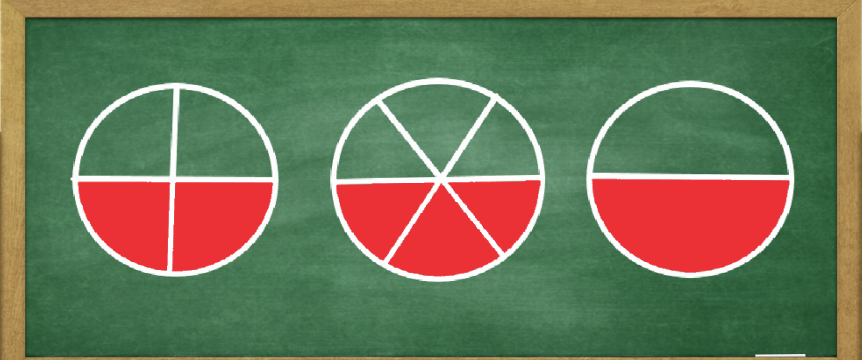
The first pie is divided into iv shares and two are shaded ruddy. But every bit we can run across that's half the pie. The second pie is divided into 6 shares and iii are shaded ruddy. Half of the pie again. Finally, the tertiary pie is divided into ii halves and one half is shaded red.
Since it's half a pie that'southward shaded in either example, nosotros can conclude that the fractions are equal: two/4 = iii/6 = i/ii.
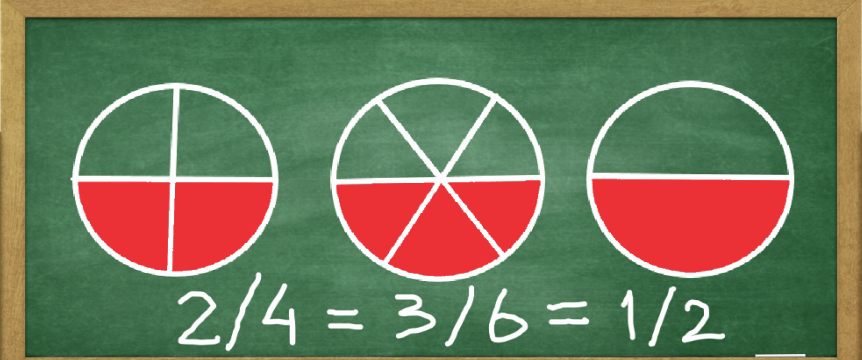
Finally, by multiplying or dividing both the numerator and denominator past the same number, the fraction will stay the same (except the case when sectionalisation is by nada, which is outside of the scope of this article and will not be considered here).
This dominion helps simplify fractions and makes using them easier. As an case, let'south consider 4/12. Dividing numerator and denominator by 4 gives u.s.a. (4 : four) / (12 : 4) = 1 / 3. It's fourth dimension to examination your knowledge.
What fraction is the aforementioned as 2/v?
Choose ane answer
Part 3. Comparing fractions
When we come across two pieces of a pie, we tin usually tell which i is larger. Similarly with fractions, there is a simple way of comparing them to each other.
Say we demand to compare 1/iii and 2/7. Since they take different denominators, they accept a different number of parts. So the Showtime step must be to find the common basis. We practice it by finding a mutual denominator.
One of the methods for finding a common denominator of two or more fractions is to multiply the denominators with each other. 3 times seven = 21.
Now that nosotros plant the common denominator, we need to replace each fraction'southward ain denominator with the common denominator.
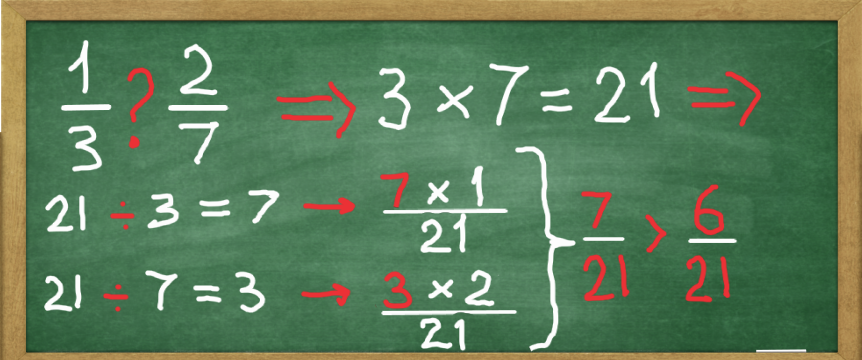
The first fraction is 1/3, so we divide 21 by three and resulting 7 gets multiplied by that fractions numerator. Since the numerator equals 1, we get seven times i = seven.
The 2d fraction is 2/vii, so 21 divided by 7 results in 3. Multiplying three times this fractions numerator, gives usa 3 times ii = 6.
At present that the fractions take the aforementioned denominator, we tin finally compare them. 7 shares is more than half-dozen shares, therefore vii/21 is greater than 6/21.
The mathematical symbol denoting our outcome is the > sign. 7/21 > 6/21. It is read equally "greater-than." The symbol denoting bottom-than looks similar this: <. We can rewrite our event like this: six/21 < 7/21.
Compare 3/iv and v/7
Cull ane answer
Office iv. Adding fractions
To add together fractions, we again need to find a mutual denominator. Let'due south look at the following example.
Nosotros need to add together 2/7 and 3/9. The common denominator is 7 times 9 = 63. The next step would exist to replace each fraction's ain denominator with the mutual one.
For the first fraction, 63 divided by 7 = 9 and 9 times 2 = 18. The issue is eighteen/63. For the second one, 63 divided by 9 = 7 and seven times 3 = 21. The consequence is 21/63.
Next, we add the numerators. 18 plus 21 = 39, which leaves usa with the sum of 39/63.
Every bit a useful habit, ever check if the resulting fraction tin be further simplified.
We know that 39 is evenly divisible by iii. 63 is also evenly divisible by 3. Since both numerator and denominator are divided by the same number, the fraction volition remain the same. 39 divided past 3 = 13 and 63 divided by 3 = 21. Our final result is 13/21.
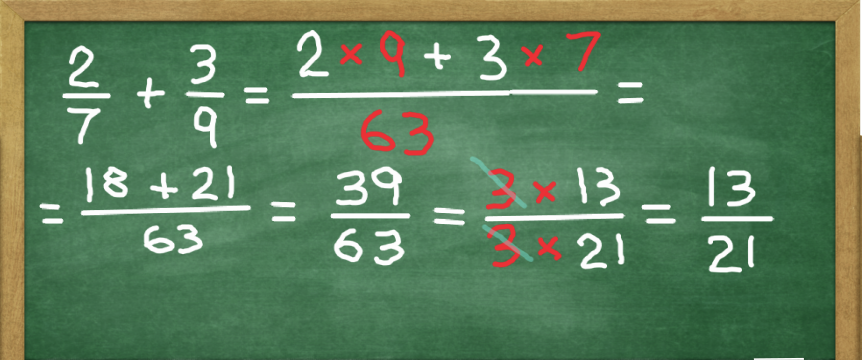
What if we need to add mixed numbers? To add together mixed numbers, nosotros get-go add the whole numbers together and so the fractions.
For example, to add i and a half to two and a half, add 1 and 2 = 3, then add ane/2 and 1/2 = 1. Finally, add 3 and 1 = 4. Let's have some practice and remember how to simplify results.
What is the upshot of 4/6 + 2/9?
Choose 1 reply
Part 5. Subtracting fractions
We'll outset with two simple fractions. Decrease ane/three from 3/5. As in the case of addition, we need to find a common denominator. So if we multiply our denominators, that equals iii times 5 = 15.
Next, we supersede onetime denominators with the mutual one.
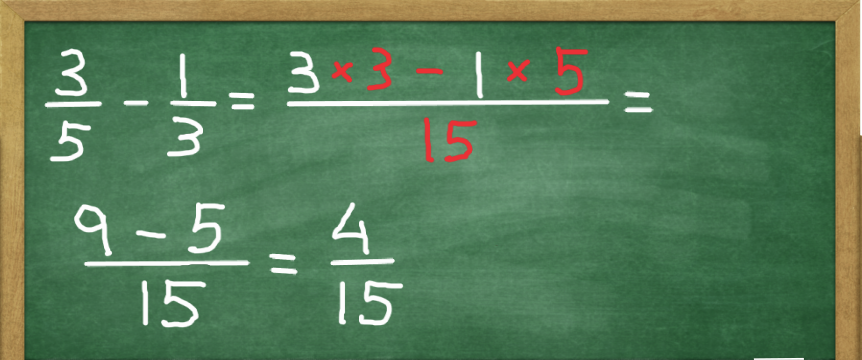
And then we need to find our numerators. For the first fraction, 15 divided past 5 = 3 and 3 times three = 9. The result is 9/fifteen. For the 2d ane, fifteen divided by 3 = 5 and 5 times 1 = 5. The result is 5/15.
The last step is to subtract the adjusted numerators: 9 minus five = four. The resulting fraction equals 4/fifteen.
Let's now look at the case when we demand to decrease a fraction from a whole number. Allow'south begin with 1 - 2/7.
Yous remember from previous sections that a whole number is like a pie that is completely shaded. Thus, if a pie is divided into 3 parts, all iii parts are shaded. If it is divided into 7 parts, and so 7 parts volition exist shaded. So, ane = three/3 = 7/7 etc.
Since we need to subtract 2/7, nosotros'll plough 1 whole into 7/vii to make our job easy. seven/7 minus 2/7 = five/seven. If the whole number is other than 1, we write it as a mixed number and follow the steps from the concluding instance.
So let'due south subtract 2/7 from 3.
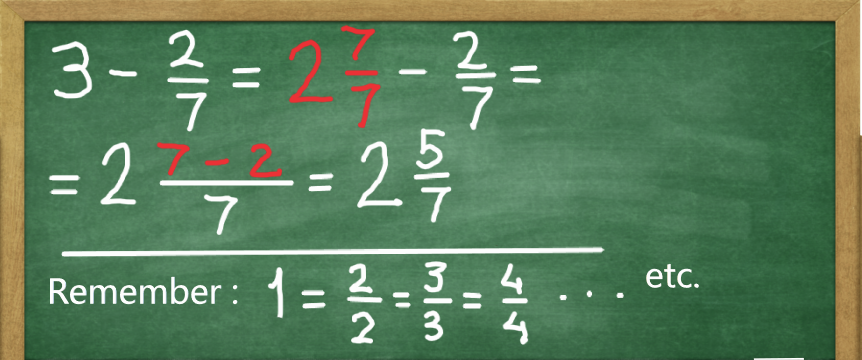
Frequently, as a result of calculations, we may finish upwardly with a fraction where the numerator is greater than or equal to the denominator. Such fractions are chosen improper fractions. For case 5/3 (five thirds), 7/2 (seven halves) and then on. They tin can be converted to mixed numbers and vice versa.
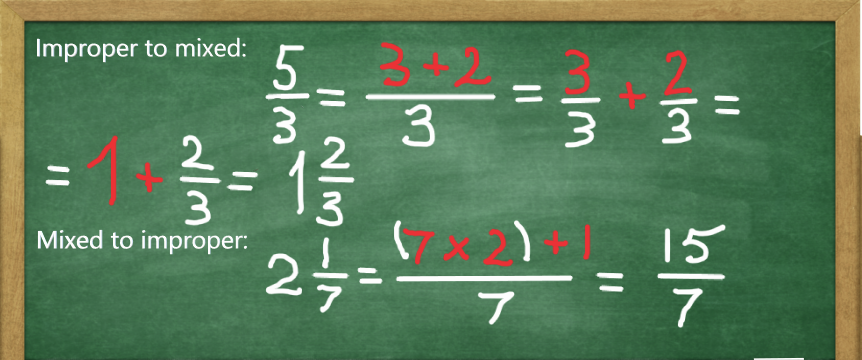
All the rules covered so far utilize to improper fractions as well.
What is the result of ix/eleven - 3/4?
Cull 1 reply
Part vi. Multiplying fractions
Suppose we demand to multiply 2 fractions, 2/5 times 3/7. The numerator of the product volition be the production of the numerators of these fractions: 2 times iii = half dozen. The denominator of the production will exist the product of denominators of these fractions: 5 times vii = 35. Thus, 2/5 times three/7 = vi/35.
If we demand to multiply a fraction by a whole number, the numerator of the product volition be the production of the numerator of the fraction and that whole number. The denominator of the product will remain the same every bit the denominator of the fraction.
For example, iii/x times 5 = 15/10. To simplify, we dissever the numerator and denominator by 5 and get 3/2.
Finally, if we need to multiply mixed numbers, first we convert them to improper fractions, so multiply them as we did above. The example below shows the steps.
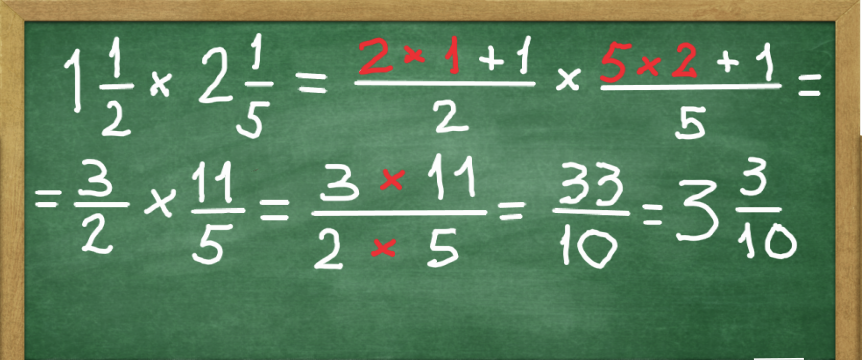
Part seven. Dividing fractions
To split fractions, flip the divisor and then its numerator becomes the new denominator and denominator becomes the new numerator. So just multiply the fractions similar we did earlier.
For case, split 3/7 by two/5. Afterward flipping, 2/5 becomes 5/2 and we stop upwards multiplying three/7 times five/2 = 15/14.
To divide fraction by a whole number, nosotros invert that number and it becomes 1 divided by that number.
For instance, 2 becomes i/2, ix becomes 1/9 etc. Next, we multiply as above. Every bit you probably guessed already, dividing mixed numbers works the same style. Let'southward look at the instance below.
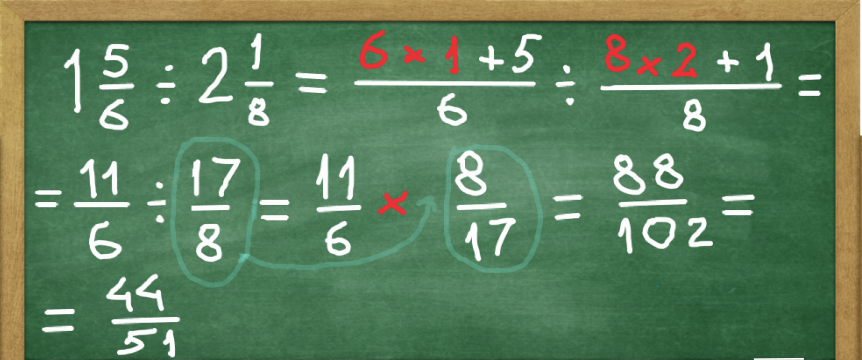
Let's exam your knowledge.
What is the result of 11/three divided past 11/vii?
Cull 1 reply
Part 8. Some applied examples
In order to find a fraction of some number, nosotros need to multiply the given number past that fraction.
Imagine, your schoolhouse textbook has 200 pages. If you read 3/5 of the textbook, how many pages take you read? Nosotros are given the number which equals 200. To discover 3/5 of 200, nosotros multiply 200 times three/five and get120 pages.
Solve the next question on your ain. My birthday cake had 12 pieces. A few friends came by and enjoyed ii/3 of the cake. How many pieces did my friends have?
How many pieces did my friends have?
Cull 1 answer
Finally, there is ane more case I desire to explore. What if we know what a given fraction of some number equals and we need to detect that number?
For example, we know my friends had viii pieces of the altogether block and that was 2/3 of the whole cake. How many pieces did the cake take in the starting time? In order to find that whole number, nosotros demand to divide 8 past 2/3, which is 12.
Solve the next question on your ain. A race car drove 900 meters on a rails, which is three/5 of the whole altitude. What's the length of the race rail?
What is the length of the race track?
Cull 1 answer
Learn to code for gratuitous. freeCodeCamp'due south open source curriculum has helped more than twoscore,000 people get jobs as developers. Become started
Source: https://www.freecodecamp.org/news/fraction-math-how-to-do-fractions-for-beginners/
Posted by: cummingstheplain.blogspot.com


0 Response to "How To Find The Domain Of A Fraction"
Post a Comment